|
|
|
|
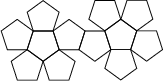
The regular dodecahedron is the Platonic Solid (![]() ) composed of 20 Vertices, 30 Edges, and 12 Pentagonal Faces. It is given by the symbol
) composed of 20 Vertices, 30 Edges, and 12 Pentagonal Faces. It is given by the symbol ![]() , the
Schläfli Symbol
, the
Schläfli Symbol ![]() . It is also Uniform Polyhedron
. It is also Uniform Polyhedron ![]() and has Wythoff
Symbol
and has Wythoff
Symbol ![]() . The dodecahedron has the Icosahedral Group
. The dodecahedron has the Icosahedral Group ![]() of symmetries.
of symmetries.
A Plane Perpendicular to a ![]() axis of a dodecahedron cuts the solid in a regular Hexagonal
Cross-Section (Holden 1991, p. 27). A Plane Perpendicular to a
axis of a dodecahedron cuts the solid in a regular Hexagonal
Cross-Section (Holden 1991, p. 27). A Plane Perpendicular to a ![]() axis of a dodecahedron cuts the
solid in a regular Decagonal Cross-Section (Holden 1991, p. 24).
axis of a dodecahedron cuts the
solid in a regular Decagonal Cross-Section (Holden 1991, p. 24).
The Dual Polyhedron of the dodecahedron is the Icosahedron.
When the dodecahedron with edge length
![]() is oriented with two opposite faces parallel to the
is oriented with two opposite faces parallel to the
![]() -Plane, the vertices of the top and bottom faces lie at
-Plane, the vertices of the top and bottom faces lie at ![]() and the other Vertices lie at
and the other Vertices lie at ![]() , where
, where ![]() is the Golden Ratio. The explicit coordinates are
is the Golden Ratio. The explicit coordinates are
| (1) |
| (2) |
| (3) | |||
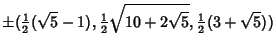 |
(4) | ||
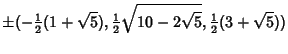 |
(5) | ||
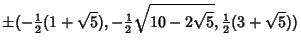 |
|||
| (6) | |||
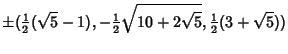 |
(7) | ||
| (8) | |||
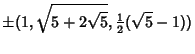 |
(9) | ||
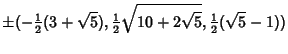 |
(10) | ||
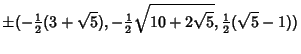 |
|||
| (11) | |||
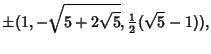 |
(12) |
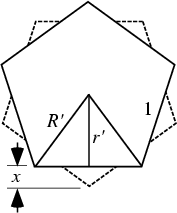
For a dodecahedron of unit edge length ![]() , the Circumradius
, the Circumradius ![]() and Inradius
and Inradius ![]() of a
Pentagonal Face are
of a
Pentagonal Face are
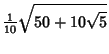 |
(13) | ||
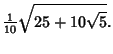 |
(14) |
| (15) |
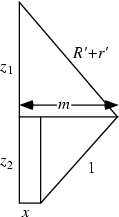
Using the Pythagorean Theorem on the figure then gives
| (16) | |||
| (17) | |||
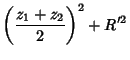 |
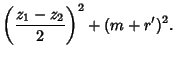 |
(18) |
| (19) |
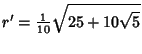 |
(20) | ||
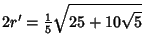 |
(21) | ||
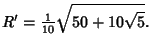 |
(22) |
| (23) |
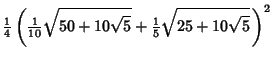 |
|||
| (24) |
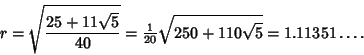 |
(25) |
| (26) |
| (27) |
| (28) |
| (29) |
The Area of a single Face is the Area of a Pentagon,
| (30) |
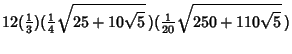 |
|||
| (31) |
| (32) |
The Hexagonal Scalenohedron is an irregular dodecahedron.
See also Augmented Dodecahedron, Augmented Truncated Dodecahedron, Dodecagon, Dodecahedron-Icosahedron Compound, Elongated Dodecahedron, Great Dodecahedron, Great Stellated Dodecahedron, Hyperbolic Dodecahedron, Icosahedron, Metabiaugmented Dodecahedron, Metabiaugmented Truncated Dodecahedron, Parabiaugmented Dodecahedron, Parabiaugmented Truncated Dodecahedron, Pyritohedron, Rhombic Dodecahedron, Small Stellated Dodecahedron, Triaugmented Dodecahedron, Triaugmented Truncated Dodecahedron, Trigonal Dodecahedron, Trigonometry Values Pi/5, Truncated Dodecahedron
References
Cundy, H. and Rollett, A. Mathematical Models, 3rd ed. Stradbroke, England: Tarquin Pub., 1989.
Davie, T. ``The Dodecahedron.''
http://www.dcs.st-and.ac.uk/~ad/mathrecs/polyhedra/dodecahedron.html.
Holden, A. Shapes, Space, and Symmetry. New York: Dover, 1991.
|
|
|
© 1996-9 Eric W. Weisstein