|
|
|

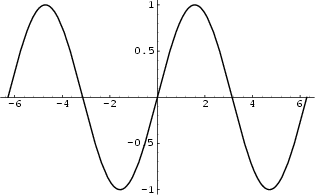
Let ![]() be an Angle measured counterclockwise from the x-Axis along the arc of the Unit Circle.
Then
be an Angle measured counterclockwise from the x-Axis along the arc of the Unit Circle.
Then ![]() is the vertical coordinate of the arc endpoint. As a result of this definition, the sine function is
periodic with period
is the vertical coordinate of the arc endpoint. As a result of this definition, the sine function is
periodic with period ![]() . By the Pythagorean Theorem,
. By the Pythagorean Theorem, ![]() also obeys the identity
also obeys the identity
| (1) |
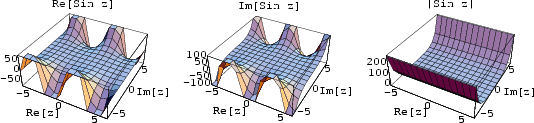
The sine function can be defined algebraically by the infinite sum
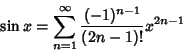 |
(2) |
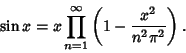 |
(3) |
| (4) |
| (5) |
Using the results from the Exponential Sum Formulas
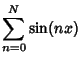 |
![$\displaystyle \Im\left[{\,\sum_{n=0}^N e^{inx}}\right]$](s1_1440.gif) |
||
![$\displaystyle \Im\left[{{\sin({\textstyle{1\over 2}}Nx)\over\sin({\textstyle{1\over 2}}x)} e^{i(N+1)x/2}}\right]$](s1_1441.gif) |
|||
![$\displaystyle {\sin({\textstyle{1\over 2}}Nx)\over\sin({\textstyle{1\over 2}}x)} \sin[{\textstyle{1\over 2}}x(N+1)].$](s1_1442.gif) |
(6) |
![\begin{displaymath}
\sum_{n=0}^\infty p^n\sin(nx) = \Im\left[{\,\sum_{n=0}^\inft...
...x}\over 1-2p\cos x+p^2}\right]= {p\sin x\over 1-2p\cos x+p^2}.
\end{displaymath}](s1_1443.gif) |
(7) |
| (8) |
| (9) |
Cvijovic and Klinowski (1995) show that the sum
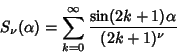 |
(10) |
| (11) |
A Continued Fraction representation of ![]() is
is
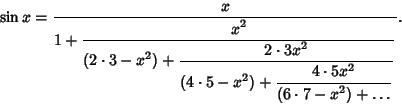 |
(12) |
The value of ![]() is Irrational for all
is Irrational for all ![]() except 4 and 12, for which
except 4 and 12, for which ![]() and
and
![]() .
.
The Fourier Transform of
![]() is given by
is given by
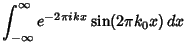 |
|||
| (13) |
Definite integrals involving ![]() include
include
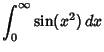 |
(14) | ||
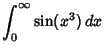 |
(15) | ||
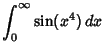 |
(16) | ||
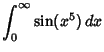 |
(17) |
See also Andrew's Sine, Cosecant, Cosine, Fourier Transform--Sine, Hyperbolic Sine, Sinc Function, Tangent, Trigonometry
References
Abramowitz, M. and Stegun, C. A. (Eds.). ``Circular Functions.'' §4.3 in
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, pp. 71-79, 1972.
Cvijovic, D. and Klinowski, J. ``Closed-Form Summation of Some Trigonometric Series.'' Math. Comput. 64, 205-210, 1995.
Hansen, E. R. A Table of Series and Products. Englewood Cliffs, NJ: Prentice-Hall, 1975.
Project Mathematics! Sines and Cosines, Parts I-III. Videotapes (28, 30, and 30 minutes). California Institute of
Technology. Available from the Math. Assoc. Amer.
Spanier, J. and Oldham, K. B. ``The Sine
![]() and Cosine
and Cosine ![]() Functions.''
Ch. 32 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 295-310, 1987.
Functions.''
Ch. 32 in An Atlas of Functions. Washington, DC: Hemisphere, pp. 295-310, 1987.
|
|
|
© 1996-9 Eric W. Weisstein