Truncated Octahedron

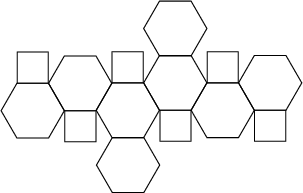
An Archimedean Solid, also known as the Mecon, whose Dual Polyhedron is the Tetrakis Hexahedron. It is also Uniform Polyhedron $U_8$ and has Schläfli Symbol t$\{3,4\}$ and Wythoff Symbol $2\,4\,\vert\,3$. The faces of the truncated octahedron are $8\{6\}+6\{4\}$. The truncated octahedron has the $O_h$ Octahedral Group of symmetries.

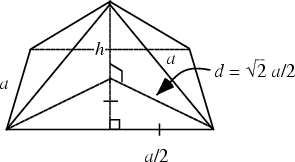
The solid can be formed from an Octahedron via Truncation by removing six Square Pyramids, each with edge slant height $a=s/3$ and height $h$, where $s$ is the side length of the original Octahedron. From the above diagram, the height and base area of the Square Pyramid are

$$h = \sqrt{a^2-d^2}={\textstyle{1\over 2}}\sqrt{2}\,a$$ (1)

$$A_b = a^2.$$ (2)

The Volume of the truncated octahedron is then given by the Volume of the Octahedron

$$V_{\rm octahedron}={\textstyle{1\over 3}}\sqrt{2}\,s^3=9\sqrt{2}\,a^3$$ (3)

minus six times the volume of the Square Pyramid,

$$V=V_{\rm octahedron}-6({\textstyle{1\over 3}}A_b h)=(9\sqrt{2}-\sqrt{2})a^3=8\sqrt{2}\,a^3.$$ (4)

The truncated octahedron is a Space-Filling Polyhedron. The Inradius, Midradius, and Circumradius for $a=1$ are

$$r = {\textstyle{9\over 20}}\sqrt{10} \approx 1.42302$$ (5)

$$\rho = {\textstyle{3\over 2}} = 1.5$$ (6)

$$R = {\textstyle{1\over 2}}\sqrt{10} \approx 1.58114.$$ (7)

See also Octahedron, Square Pyramid, Truncation

References

Coxeter, H. S. M. Regular Polytopes, 3rd ed. New York: Dover, pp. 29-30 and 257, 1973.