|
|
|

An obtuse triangle is a Triangle in which one of the Angles is an Obtuse Angle. (Obviously, only a single Angle in a Triangle can be Obtuse or it wouldn't be a Triangle.) A triangle must be either obtuse, Acute, or Right.
A famous problem is to find the chance that three points picked randomly in a Plane are the Vertices of an obtuse triangle (Eisenberg and Sullivan 1996). Unfortunately, the solution of the problem depends on the
procedure used to pick the ``random'' points (Portnoy 1994). In fact, it is impossible to pick random variables which are
uniformly distributed in the plane (Eisenberg and Sullivan 1996). Guy (1993) gives a variety of solutions to the problem.
Woolhouse (1886) solved the problem by picking uniformly distributed points in the unit Disk, and obtained
| (1) |
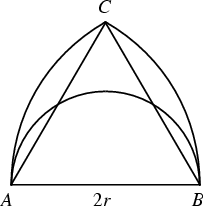
Lewis Carroll (1893) posed and gave another solution to the problem as follows. Call the longest side of a Triangle
![]() , and call the Diameter
, and call the Diameter ![]() . Draw arcs from
. Draw arcs from ![]() and
and ![]() of Radius
of Radius ![]() . Because the longest side of the
Triangle is defined to be
. Because the longest side of the
Triangle is defined to be ![]() , the third Vertex of the Triangle must lie within
the region
, the third Vertex of the Triangle must lie within
the region ![]() . If the third Vertex lies within the Semicircle, the Triangle is
an obtuse triangle. If the Vertex lies on the Semicircle (which will happen with
probability 0), the Triangle is a Right Triangle. Otherwise, it is an Acute Triangle. The chance of
obtaining an obtuse triangle is then the ratio of the Area of the Semicircle to that of
. If the third Vertex lies within the Semicircle, the Triangle is
an obtuse triangle. If the Vertex lies on the Semicircle (which will happen with
probability 0), the Triangle is a Right Triangle. Otherwise, it is an Acute Triangle. The chance of
obtaining an obtuse triangle is then the ratio of the Area of the Semicircle to that of ![]() . The
Area of
. The
Area of ![]() is then twice the Area of a Sector minus the Area of the Triangle.
is then twice the Area of a Sector minus the Area of the Triangle.
| (2) |
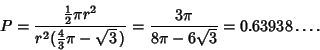 |
(3) |
Let the Vertices of a triangle in ![]() -D be Normal
(Gaussian) variates. The probability that a Gaussian triangle in
-D be Normal
(Gaussian) variates. The probability that a Gaussian triangle in ![]() -D is obtuse is
-D is obtuse is
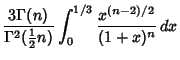 |
|||
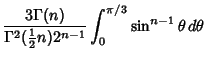 |
|||
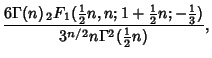 |
(4) |
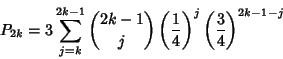 |
(5) |
| (6) | |||
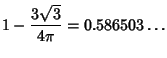 |
(7) | ||
| (8) | |||
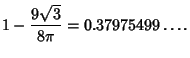 |
(9) |
See also Acute Angle, Acute Triangle, Ball Triangle Picking, Obtuse Angle, Right Triangle, Triangle
References
Buchta, C. ``A Note on the Volume of a Random Polytope in a Tetrahedron.'' Ill. J. Math. 30, 653-659, 1986.
Carroll, L. Pillow Problems & A Tangled Tale. New York: Dover, 1976.
Eisenberg, B. and Sullivan, R. ``Random Triangles
Guy, R. K. ``There are Three Times as Many Obtuse-Angled Triangles as There are Acute-Angled Ones.'' Math. Mag.
66, 175-178, 1993.
Hall, G. R. ``Acute Triangles in the
Portnoy, S. ``A Lewis Carroll Pillow Problem: Probability on at Obtuse Triangle.'' Statist. Sci. 9, 279-284, 1994.
Wells, D. G. The Penguin Book of Interesting Puzzles. London: Penguin Books, pp. 67 and 248-249, 1992.
Woolhouse, W. S. B. Solution to Problem 1350. Mathematical Questions, with Their Solutions, from the Educational Times, 1.
London: F. Hodgson and Son, 49-51, 1886.
![]() Dimensions.'' Amer. Math. Monthly 103, 308-318, 1996.
Dimensions.'' Amer. Math. Monthly 103, 308-318, 1996.
![]() -Ball.'' J. Appl. Prob. 19, 712-715, 1982.
-Ball.'' J. Appl. Prob. 19, 712-715, 1982.
|
|
|
© 1996-9 Eric W. Weisstein