|
|
|
|
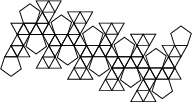
An Archimedean Solid, also called the Snub Icosidodecahedron, whose Dual Polyhedron is the
Pentagonal Hexecontahedron. It has Schläfli Symbol s
![]() . It is
also Uniform Polyhedron
. It is
also Uniform Polyhedron ![]() and has Wythoff Symbol
and has Wythoff Symbol ![]() . Its faces are
. Its faces are
![]() . For
. For
![]() , it has Inradius, Midradius, and Circumradius
, it has Inradius, Midradius, and Circumradius
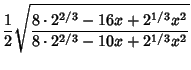 |
|||
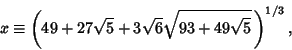
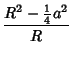 |
|||
References
Coxeter, H. S. M.; Longuet-Higgins, M. S.; and Miller, J. C. P. ``Uniform Polyhedra.''
Phil. Trans. Roy. Soc. London Ser. A 246, 401-450, 1954.