|
|
|
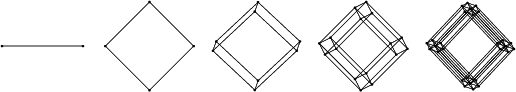
The generalization of a 3-Cube to ![]() -D, also called a Measure Polytope. It is a regular Polytope
with mutually Perpendicular sides, and is therefore an Orthotope. It is denoted
-D, also called a Measure Polytope. It is a regular Polytope
with mutually Perpendicular sides, and is therefore an Orthotope. It is denoted ![]() and has
Schläfli Symbol
and has
Schläfli Symbol
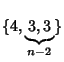 . The number of
. The number of ![]() -cubes
contained in an
-cubes
contained in an ![]() -cube can be found from the Coefficients of
-cube can be found from the Coefficients of ![]() .
.
|
The 1-hypercube is a Line Segment, the 2-hypercube is the Square, and the 3-hypercube is the Cube. The
hypercube in ![]() , called a Tesseract, has the Schläfli Symbol
, called a Tesseract, has the Schläfli Symbol ![]() and
Vertices
and
Vertices
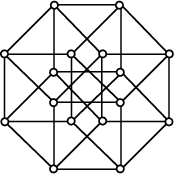
![]() . The above figures show two visualizations of the
Tesseract. The figure on the left is a projection of the Tesseract in 3-space (Gardner 1977), and the figure on
the right is the Graph of the Tesseract symmetrically projected into the Plane
(Coxeter 1973). A Tesseract has 16 Vertices, 32 Edges,
24 Squares, and eight Cubes.
. The above figures show two visualizations of the
Tesseract. The figure on the left is a projection of the Tesseract in 3-space (Gardner 1977), and the figure on
the right is the Graph of the Tesseract symmetrically projected into the Plane
(Coxeter 1973). A Tesseract has 16 Vertices, 32 Edges,
24 Squares, and eight Cubes.
See also Cross Polytope, Cube, Hypersphere, Orthotope, Parallelepiped, Polytope, Simplex, Tesseract
References
Coxeter, H. S. M. Regular Polytopes, 3rd ed. New York: Dover, p. 123, 1973.
Gardner, M. ``Hypercubes.'' Ch. 4 in
Mathematical Carnival: A New Round-Up of Tantalizers and Puzzles from Scientific American.
New York: Vintage Books, 1977.
Geometry Center. ``The Tesseract (or Hypercube).''
http://www.geom.umn.edu/docs/outreach/4-cube/.
Pappas, T. ``How Many Dimensions are There?''
The Joy of Mathematics. San Carlos, CA: Wide World Publ./Tetra, pp. 204-205, 1989.