|
|
|
The Product of the Squares of the differences of the Polynomial Roots
![]() . For a Polynomial of degree
. For a Polynomial of degree ![]() ,
,
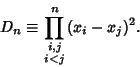 |
(1) |
The discriminant of the Quadratic Equation
| (2) |
| (3) |
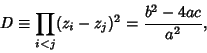 |
(4) |
The discriminant of the Cubic Equation
| (5) |
| (6) |
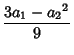 |
(7) | ||
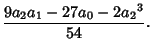 |
(8) |
| (9) |
| (10) |
| (11) |
The discriminant of a Quartic Equation
| (12) |
|
|
|
|
|
|
|
|
(13) |
See also Resultant
References
Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, Feb. 1972.
|
|
|
© 1996-9 Eric W. Weisstein