|
|
|
A Quartic Nonorientable Surface, also known as the Steiner Surface. The Roman surface is one of the three possible surfaces obtained by sewing a Möbius Strip to the edge of a Disk. The other two are the Boy Surface and Cross-Cap, all of which are homeomorphic to the Real Projective Plane (Pinkall 1986).
The center point of the Roman surface is an ordinary Triple Point with
![]() ,
and the six endpoints of the three lines of self-intersection are singular Pinch Points, also known as
Whitney Singularities. The Roman surface is essentially six Cross-Caps
stuck together and contains a double Infinity of Conics.
,
and the six endpoints of the three lines of self-intersection are singular Pinch Points, also known as
Whitney Singularities. The Roman surface is essentially six Cross-Caps
stuck together and contains a double Infinity of Conics.
The Roman surface can given by the equation
| (1) |
| (2) |
![\begin{displaymath}
{\hbox{\sf R}}_z(45^\circ)={1\over\sqrt{2}}\left[{\matrix{1 & 1 & 0\cr -1 & 1 & 0\cr 0 & 0 & 1\cr}}\right]
\end{displaymath}](r_1934.gif) |
(3) |
![\begin{displaymath}
\left[{\matrix{x'\cr y'\cr z'\cr}}\right] = {\hbox{\sf R}}_z(45^\circ)\left[{\matrix{x\cr y\cr z\cr}}\right],
\end{displaymath}](r_1935.gif) |
(4) |
| (5) |
| (6) |
| (7) | |||
| (8) | |||
| (9) |
| (10) | |||
| (11) | |||
| (12) |
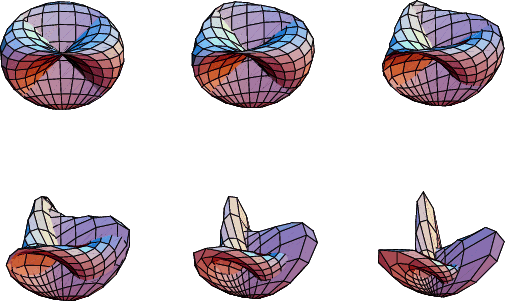
A Homotopy (smooth deformation) between the Roman surface and Boy Surface is given by the equations
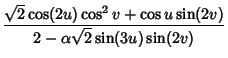 |
(13) | ||
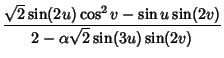 |
(14) | ||
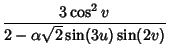 |
(15) |
See also Boy Surface, Cross-Cap, Heptahedron, Möbius Strip, Nonorientable Surface, Quartic Surface, Steiner Surface
References
Fischer, G. (Ed.). Mathematical Models from the Collections of Universities and Museums.
Braunschweig, Germany: Vieweg, p. 19, 1986.
Fischer, G. (Ed.). Plates 42-44 and 108-114 in
Mathematische Modelle/Mathematical Models, Bildband/Photograph Volume.
Braunschweig, Germany: Vieweg, pp. 42-44 and 108-109, 1986.
Geometry Center. ``The Roman Surface.''
http://www.geom.umn.edu/zoo/toptype/pplane/roman/.
Gray, A. Modern Differential Geometry of Curves and Surfaces.
Boca Raton, FL: CRC Press, pp. 242-243, 1993.
Nordstrand, T. ``Steiner's Roman Surface.''
http://www.uib.no/people/nfytn/steintxt.htm.
Pinkall, U. Mathematical Models from the Collections of Universities and Museums (Ed. G. Fischer).
Braunschweig, Germany: Vieweg, p. 64, 1986.
Wang, P. ``Renderings.''
http://www.ugcs.caltech.edu/~peterw/portfolio/renderings/.
|
|
|
© 1996-9 Eric W. Weisstein