Neile's Parabola

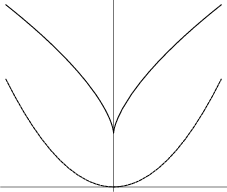
$\begin{figure}\begin{center}\BoxedEPSF{neils_parabola.epsf scaled 800}\end{center}\end{figure}$

The solid curve in the above figure which is the Evolute of the Parabola (dashed curve). In Cartesian Coordinates,

$\begin{displaymath} y={\textstyle{3\over 4}} (2x)^{2/3}+{\textstyle{1\over 2}}. \end{displaymath}$

Neile's parabola is also called the Semicubical Parabola, and was discovered by William Neile in 1657. It was the first nontrivial Algebraic Curve to have its Arc Length computed. Wallis published the method in 1659, giving Neile the credit (MacTutor Archive).

See also Parabola Evolute

References

Lee, X. ``Semicubic Parabola.'' http://www.best.com/~xah/SpecialPlaneCurves_dir/SemicubicParabola_dir/semicubicParabola.html

MacTutor History of Mathematics Archive. ``Neile's Semi-Cubical Parabola.'' http://www-groups.dcs.st-and.ac.uk/~history/Curves/Neiles.html.