|
|
|
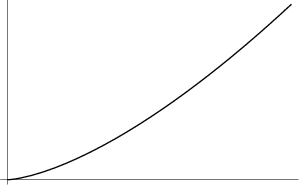
A Parabola-like curve with Cartesian equation
| (1) |
| (2) | |||
| (3) |
| (4) |
The Arc Length, Curvature, and Tangential Angle are
| (5) | |||
| (6) | |||
| (7) |
See also Neile's Parabola, Parabola Involute
References
Gray, A. ``The Semicubical Parabola.'' §1.7 in
Modern Differential Geometry of Curves and Surfaces. Boca Raton, FL: CRC Press, pp. 15-16, 1993.
Lawrence, J. D. A Catalog of Special Plane Curves. New York: Dover, pp. 85-87, 1972.
Lee, X. ``Semicubic Parabola.''
http://www.best.com/~xah/SpecialPlaneCurves_dir/SemicubicParabola_dir/semicubicParabola.html
MacTutor History of Mathematics Archive. ``Neile's Parabola.''
http://www-groups.dcs.st-and.ac.uk/~history/Curves/Neiles.html.
Yates, R. C. ``Semi-Cubic Parabola.'' A Handbook on Curves and Their Properties. Ann Arbor, MI: J. W. Edwards, pp. 186-187, 1952.