|
|
|
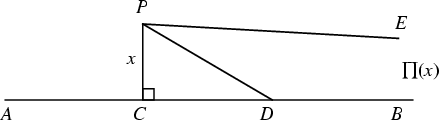
Given a point ![]() and a Line
and a Line ![]() , draw the Perpendicular through
, draw the Perpendicular through ![]() and call it
and call it ![]() . Let
. Let ![]() be any other
line from
be any other
line from ![]() which meets
which meets ![]() in
in ![]() . In a Hyperbolic Geometry, as
. In a Hyperbolic Geometry, as ![]() moves off to infinity along
moves off to infinity along ![]() , then the
line
, then the
line ![]() approaches the limiting line
approaches the limiting line ![]() , which is said to be parallel to
, which is said to be parallel to ![]() at
at ![]() . The angle
. The angle ![]() which
which ![]() makes with
makes with ![]() is then called the Angle of Parallelism for perpendicular distance
is then called the Angle of Parallelism for perpendicular distance ![]() , and is given by
, and is given by
See also Angle of Parallelism, Hyperbolic Geometry
References
Manning, H. P. Introductory Non-Euclidean Geometry. New York: Dover, p. 58, 1963.