|
|
|
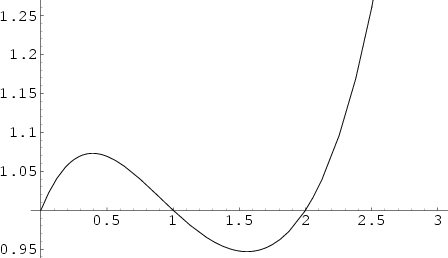

Defined in Whittaker and Watson (1990, p. 264) and also called the Barnes G-Function.
![\begin{displaymath}
G(z+1)\equiv (2\pi)^{z/2} e^{-[z(z+1)+\gamma z^2]/2}\prod_{n...
... \left[{\left({1+{z\over n}}\right)^n e^{-z+z^2/(2n)}}\right],
\end{displaymath}](g_3.gif) |
(1) |
| (2) |
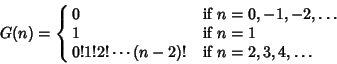 |
(3) |
The ![]() -function is the reciprocal of the Double Gamma Function. It satisfies
-function is the reciprocal of the Double Gamma Function. It satisfies
| (4) |
| (5) |
| (6) |
| (7) |
| (8) | |||
| (9) |
| (10) |
An unrelated pair of functions are denoted ![]() and
and ![]() and are known as Ramanujan g- and G-Functions.
and are known as Ramanujan g- and G-Functions.
See also Euler-Mascheroni Constant, Glaisher-Kinkelin Constant, K-Function, Meijer's G-Function, Ramanujan g- and G-Functions, Superfactorial
References
Barnes, E. W. ``The Theory of the
Glaisher, J. W. L. ``On a Numerical Continued Product.'' Messenger Math. 6, 71-76, 1877.
Glaisher, J. W. L. ``On the Product
Glaisher, J. W. L. ``On Certain Numerical Products.'' Messenger Math. 23, 145-175, 1893.
Glaisher, J. W. L. ``On the Constant which Occurs in the Formula for
Kinkelin. ``Über eine mit der Gammafunktion verwandte Transcendente und deren Anwendung auf die Integralrechnung.''
J. Reine Angew. Math. 57, 122-158, 1860.
Sloane, N. J. A. Sequence
A000178/M2049
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.
Voros, A. ``Spectral Functions, Special Functions and the Selberg Zeta Function.'' Commun. Math. Phys. 110, 439-465, 1987.
Whittaker, E. T. and Watson, G. N. A Course in Modern Analysis, 4th ed. Cambridge, England:
Cambridge University Press, 1990.
![]() -Function.'' Quart. J. Pure Appl. Math. 31, 264-314, 1900.
-Function.'' Quart. J. Pure Appl. Math. 31, 264-314, 1900.
![]() .'' Messenger Math. 7, 43-47, 1878.
.'' Messenger Math. 7, 43-47, 1878.
![]() .'' Messenger Math. 24, 1-16, 1894.
.'' Messenger Math. 24, 1-16, 1894.
|
|
|
© 1996-9 Eric W. Weisstein