|
|
|
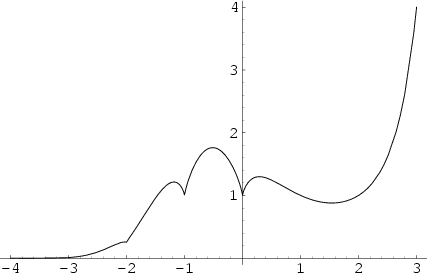
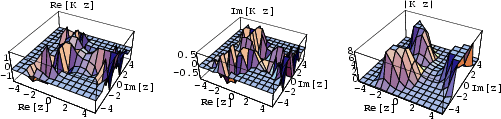
An extension of the ![]() -function
-function
| (1) |
| (2) |
| (3) |
The ![]() -function is given by the integral
-function is given by the integral
| (4) |
| (5) |
| (6) |
| (7) |
| (8) | |||
| (9) | |||
| (10) |
The first few values of ![]() for
for ![]() , 3, ... are 1, 4, 108, 27648, 86400000, 4031078400000, ... (Sloane's A002109).
These numbers are called Hyperfactorials by Sloane and Plouffe (1995).
, 3, ... are 1, 4, 108, 27648, 86400000, 4031078400000, ... (Sloane's A002109).
These numbers are called Hyperfactorials by Sloane and Plouffe (1995).
See also G-Function, Glaisher-Kinkelin Constant, Hyperfactorial, Stirling's Series
References
Sloane, N. J. A. Sequence
A002109/M3706
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.
|
|
|
© 1996-9 Eric W. Weisstein