|
|
|
N.B. A detailed on-line essay by S. Finch was the starting point for this entry.
Define
| (1) | |||
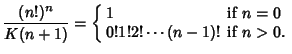 |
|||
| (2) |
| (3) |
| (4) |
| (5) |
![\begin{displaymath}
A=2^{7/36}\pi^{-1/6}\mathop{\rm exp}\nolimits \left\{{{1\over 3}+{2\over 3}\int_0^{1/2} \ln[\Gamma(x+1)]\,dx}\right\}.
\end{displaymath}](g_1506.gif) |
(6) |
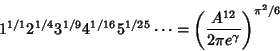 |
(7) |
 |
(8) |
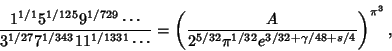 |
(9) |
| (10) |
See also G-Function, Hyperfactorial, K-Function
References
Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/glshkn/glshkn.html
Glaisher, J. W. L. ``On a Numerical Continued Product.'' Messenger Math. 6, 71-76, 1877.
Glaisher, J. W. L. ``On the Product
Glaisher, J. W. L. ``On Certain Numerical Products.'' Messenger Math. 23, 145-175, 1893.
Glaisher, J. W. L. ``On the Constant which Occurs in the Formula for
Kinkelin. ``Über eine mit der Gammafunktion verwandte Transcendente und deren Anwendung auf die Integralrechnung.''
J. Reine Angew. Math. 57, 122-158, 1860.
![]() .'' Messenger Math. 7, 43-47, 1878.
.'' Messenger Math. 7, 43-47, 1878.
![]() .'' Messenger Math.
24, 1-16, 1894.
.'' Messenger Math.
24, 1-16, 1894.
|
|
|
© 1996-9 Eric W. Weisstein