|
|
|
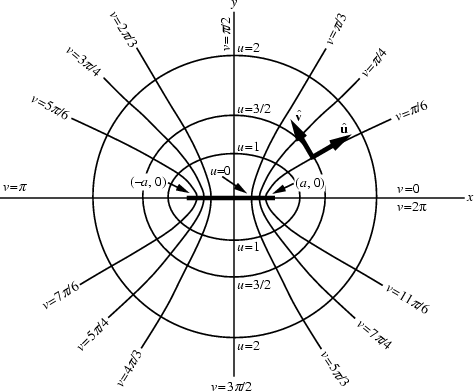
The ![]() coordinates are the asymptotic angle of confocal Parabola segments symmetrical about the
coordinates are the asymptotic angle of confocal Parabola segments symmetrical about the ![]() axis. The
axis. The
![]() coordinates are confocal Ellipses centered on the origin.
coordinates are confocal Ellipses centered on the origin.
| (1) | |||
| (2) | |||
| (3) |
| (4) |
| (5) |
| (6) | |||
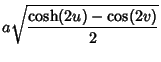 |
(7) | ||
| (8) | |||
| (9) | |||
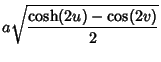 |
(10) | ||
| (11) | |||
| (12) |
| (13) |
| (14) | |||
| (15) | |||
| (16) |
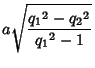 |
(17) | ||
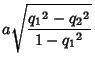 |
(18) | ||
| (19) |
See also Cylindrical Coordinates, Helmholtz Differential Equation--Elliptic Cylindrical Coordinates
References
Arfken, G. ``Elliptic Cylindrical Coordinates (
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York: McGraw-Hill, p. 657, 1953.
![]() ,
, ![]() ,
, ![]() ).'' §2.7 in
Mathematical Methods for Physicists, 2nd ed. Orlando, FL: Academic Press, pp. 95-97, 1970.
).'' §2.7 in
Mathematical Methods for Physicists, 2nd ed. Orlando, FL: Academic Press, pp. 95-97, 1970.
|
|
|
© 1996-9 Eric W. Weisstein