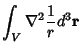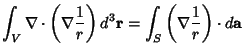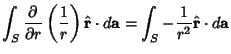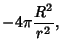The Laplacian operator for a Scalar function 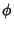 is defined by
is defined by
![\begin{displaymath}
\nabla^2 \phi = {1\over h_1h_2h_3}\left[{{\partial \over \pa...
...h_2\over h_3}{\partial \over \partial u_3}}\right)}\right]\phi
\end{displaymath}](l1_741.gif) |
(1) |
in Vector notation, where the 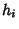 are the Scale Factors
of the coordinate system. In Tensor notation, the Laplacian is written
are the Scale Factors
of the coordinate system. In Tensor notation, the Laplacian is written
where 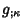 is a Covariant Derivative and
is a Covariant Derivative and
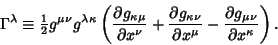 |
(3) |
The finite difference form is
For a pure radial function 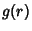 ,
,
Using the Vector Derivative identity
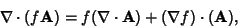 |
(6) |
so
Therefore, for a radial Power law,
A Vector Laplacian can also be defined for a Vector A by
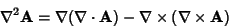 |
(9) |
in vector notation. In tensor notation, A is written 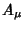 , and the identity becomes
, and the identity becomes
Similarly, a Tensor Laplacian can be given by
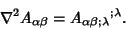 |
(11) |
An identity satisfied by the Laplacian is
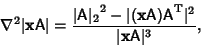 |
(12) |
where
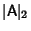 is the Hilbert-Schmidt Norm,
is the Hilbert-Schmidt Norm, 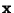 is a row Vector, and
is a row Vector, and
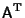 is the
Matrix Transpose of A.
is the
Matrix Transpose of A.
To compute the Laplacian of the inverse distance function 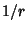 , where
, where
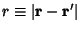 , and integrate the
Laplacian over a volume,
, and integrate the
Laplacian over a volume,
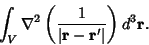 |
(13) |
This is equal to
where the integration is over a small Sphere of Radius 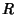 . Now, for
. Now, for 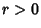 and
and 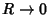 , the integral
becomes 0. Similarly, for
, the integral
becomes 0. Similarly, for 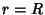 and
and 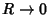 , the integral becomes
, the integral becomes 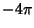 . Therefore,
. Therefore,
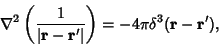 |
(15) |
where
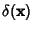 is the Delta Function.
is the Delta Function.
See also Antilaplacian
© 1996-9 Eric W. Weisstein
1999-05-26
![]() is defined by
is defined by
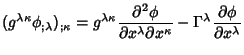
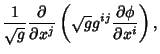
![$\displaystyle \nabla \cdot \left[{{\partial g(r)\over \partial r}\hat {\bf r}
+...
...r\sin \theta}{\partial g(r)\over \partial \phi}\hat {\boldsymbol{\phi}}}\right]$](l1_753.gif)
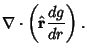
![$\displaystyle \nabla \cdot [\nabla g(r)]
= {dg\over dr}\nabla \cdot \hat {\bf r}+ \nabla \left({dg\over dr}\right)\cdot \hat {\bf r}$](l1_756.gif)
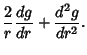
![]() , where
, where
![]() , and integrate the
Laplacian over a volume,
, and integrate the
Laplacian over a volume,