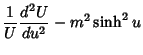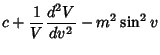In Elliptic Cylindrical Coordinates, the Scale Factors are
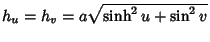 ,
, 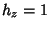 , and the separation functions are
, and the separation functions are
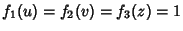 , giving a
Stäckel Determinant of
, giving a
Stäckel Determinant of
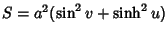 . The Helmholtz differential
equation is
. The Helmholtz differential
equation is
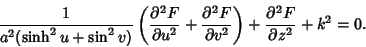 |
(1) |
Attempt Separation of Variables by writing
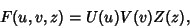 |
(2) |
then the Helmholtz Differential Equation becomes
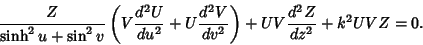 |
(3) |
Now divide by 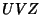 to give
to give
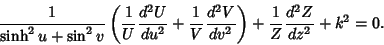 |
(4) |
Separating the 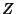 part,
part,
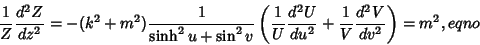 |
(5) |
so
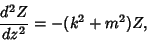 |
(6) |
which has the solution
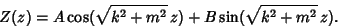 |
(7) |
Rewriting (5) gives
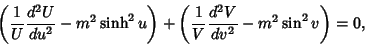 |
(8) |
which can be separated into
so
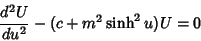 |
(11) |
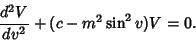 |
(12) |
Now use
![\begin{displaymath}
\sinh^2 u={\textstyle{1\over 2}}[1-\cosh(2u)]
\end{displaymath}](h_1035.gif) |
(13) |
![\begin{displaymath}
\sin^2 v={\textstyle{1\over 2}}[1-\cos(2v)]
\end{displaymath}](h_1036.gif) |
(14) |
to obtain
![\begin{displaymath}
{d^2U\over du^2}-\{c+{\textstyle{1\over 2}}m^2[1-\cosh(2u)]\}U=0
\end{displaymath}](h_1037.gif) |
(15) |
![\begin{displaymath}
{d^2V\over dv^2}+\{c+{\textstyle{1\over 2}}m^2[1-\cos(2v)]\}V=0.
\end{displaymath}](h_1038.gif) |
(16) |
Regrouping gives
![\begin{displaymath}
{d^2U\over du^2}-[(c+{\textstyle{1\over 2}}m^2)-{\textstyle{1\over 4}}m^22\cosh(2u)]U=0
\end{displaymath}](h_1039.gif) |
(17) |
![\begin{displaymath}
{d^2V\over dv^2}+[(c+{\textstyle{1\over 2}}m^2)-{\textstyle{1\over 4}}m^22\cos(2v)]V=0.
\end{displaymath}](h_1040.gif) |
(18) |
Let
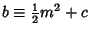 and
and
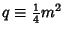 , then these become
, then these become
![\begin{displaymath}
{d^2U\over du^2}-[b-2q\cosh(2u)]U=0
\end{displaymath}](h_1043.gif) |
(19) |
![\begin{displaymath}
{d^2V\over dv^2}+[b-2q\cos(2v)]V=0.
\end{displaymath}](h_1044.gif) |
(20) |
Here, (20) is the Mathieu Differential Equation and (19) is the modified Mathieu Differential
Equation. These solutions are known as Mathieu Functions.
See also Elliptic Cylindrical Coordinates, Mathieu Differential Equation, Mathieu Function
References
Morse, P. M. and Feshbach, H. Methods of Theoretical Physics, Part I. New York:
McGraw-Hill, pp. 514 and 657, 1953.
© 1996-9 Eric W. Weisstein
1999-05-25
![]() ,
, ![]() , and the separation functions are
, and the separation functions are
![]() , giving a
Stäckel Determinant of
, giving a
Stäckel Determinant of
![]() . The Helmholtz differential
equation is
. The Helmholtz differential
equation is