Circle Lattice Points

For every Positive Integer $n$, there exists a Circle which contains exactly $n$ lattice points in its interior. H. Steinhaus proved that for every Positive Integer $n$, there exists a Circle of Area $n$ which contains exactly $n$ lattice points in its interior.

Schinzel's Theorem shows that for every Positive Integer $n$, there exists a Circle in the Plane having exactly $n$ Lattice Points on its Circumference. The theorem also explicitly identifies such ``Schinzel Circles'' as

$$\cases{ (x-{\textstyle{1\over 2}})^2+y^2={\textstyle{1\over... ...r 3}})^2+y^2={\textstyle{1\over 9}} 5^{2k} & for $n=2k+1$.\cr}$$ (1)

Note, however, that these solutions do not necessarily have the smallest possible Radius. For example, while the Schinzel Circle centered at (1/3, 0) and with Radius 625/3 has nine lattice points on its Circumference, so does the Circle centered at (1/3, 0) with Radius 65/3.

Let $r$ be the smallest Integer Radius of a Circle centered at the Origin (0, 0) with $L(r)$ Lattice Points. In order to find the number of lattice points of the Circle, it is only necessary to find the number in the first octant, i.e., those with $0\leq y\leq\left\lfloor{r/\sqrt{2}}\right\rfloor$, where $\left\lfloor{z}\right\rfloor$ is the Floor Function. Calling this $N(r)$, then for $r\geq 1$, $L(r)=8N(r)-4$, so $L(r)\equiv 4\ \left({{\rm mod\ } {8}}\right)$. The multiplication by eight counts all octants, and the subtraction by four eliminates points on the axes which the multiplication counts twice. (Since $\sqrt{2}$ is Irrational, the Midpoint of a are is never a Lattice Point.)

Gauss's Circle Problem asks for the number of lattice points within a Circle of Radius $r$

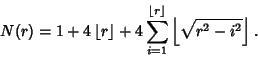$$N(r)=1+4\left\lfloor{r}\right\rfloor +4\sum_{i=1}^{\left\lfloor{r}\right\rfloor }\left\lfloor{\sqrt{r^2-i^2}}\right\rfloor .$$ (2)

Gauß showed that

$$N(r)=\pi r^2+E(r),$$ (3)

where

$$\vert E(r)\vert \leq 2\sqrt{2}\,\pi r.$$ (4)

The number of lattice points on the Circumference of circles centered at (0, 0) with radii 0, 1, 2, ... are 1, 4, 4, 4, 4, 12, 4, 4, 4, 4, 12, 4, 4, ... (Sloane's A046109). The following table gives the smallest Radius $r\leq 368,200$ for a circle centered at (0, 0) having a given number of Lattice Points $L(r)$. Note that the high water mark radii are always multiples of five.

$L(r)$	$r$	$L(r)$	$r$
1	0	108	1,105
4	1	132	40,625
12	5	140	21,125
20	25	156	203,125
28	125	180	5,525
36	65	196	274,625
44	3,125	252	27,625
52	15,625	300	71,825
60	325	324	32,045
68	390,625	420	359,125
76	$\leq 1,953,125$	540	160,225
84	1,625
92	$\leq 48,828,125$
100	4,225

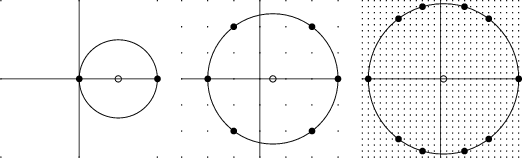
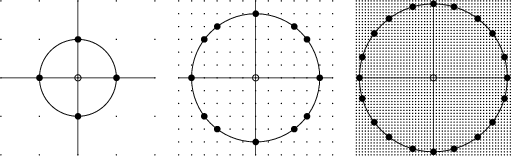
If the Circle is instead centered at (1/2, 0), then the Circles of Radii 1/2, 3/2, 5/2, ... have 2, 2, 6, 2, 2, 2, 6, 6, 6, 2, 2, 2, 10, 2, ... (Sloane's A046110) on their Circumferences. If the Circle is instead centered at (1/3, 0), then the number of lattice points on the Circumference of the Circles of Radius 1/3, 2/3, 4/3, 5/3, 7/3, 8/3, ... are 1, 1, 1, 3, 1, 1, 3, 1, 3, 1, 1, 3, 1, 3, 1, 1, 5, 3, ... (Sloane's A046111).

Let

1. $a_n$ be the Radius of the Circle centered at (0, 0) having $8n+4$ lattice points on its Circumference,

2. $b_n/2$ be the Radius of the Circle centered at (1/2, 0) having $4n+2$ lattice points on its Circumference,

3. $c_n/3$ be the Radius of Circle centered at (1/3, 0) having $2n+1$ lattice points on its Circumference.

Then the sequences $\{a_n\}$, $\{b_n\}$, and $\{c_n\}$ are equal, with the exception that $b_n=0$ if $2\vert n$ and $c_n=0$ if $3\vert n$. However, the sequences of smallest radii having the above numbers of lattice points are equal in the three cases and given by 1, 5, 25, 125, 65, 3125, 15625, 325, ... (Sloane's A046112).

Kulikowski's Theorem states that for every Positive Integer $n$, there exists a 3-D Sphere which has exactly $n$ Lattice Points on its surface. The Sphere is given by the equation

$$(x-a)^2+(y-b)^2+(z-\sqrt{2}\,)^2=c^2+2,$$

where $a$ and $b$ are the coordinates of the center of the so-called Schinzel Circle and $c$ is its Radius (Honsberger 1973).

See also Circle, Circumference, Gauss's Circle Problem, Kulikowski's Theorem, Lattice Point, Schinzel Circle, Schinzel's Theorem

References

Honsberger, R. ``Circles, Squares, and Lattice Points.'' Ch. 11 in Mathematical Gems I. Washington, DC: Math. Assoc. Amer., pp. 117-127, 1973.

Kulikowski, T. ``Sur l'existence d'une sphère passant par un nombre donné aux coordonnées entières.'' L'Enseignement Math. Ser. 2 5, 89-90, 1959.

Schinzel, A. ``Sur l'existence d'un cercle passant par un nombre donné de points aux coordonnées entières.'' L'Enseignement Math. Ser. 2 4, 71-72, 1958.

Sierpinski, W. ``Sur quelques problèmes concernant les points aux coordonnées entières.'' L'Enseignement Math. Ser. 2 4, 25-31, 1958.

Sierpinski, W. ``Sur un problème de H. Steinhaus concernant les ensembles de points sur le plan.'' Fund. Math. 46, 191-194, 1959.

Sierpinski, W. A Selection of Problems in the Theory of Numbers. New York: Pergamon Press, 1964.

Weisstein, E. W. ``Circle Lattice Points.'' Mathematica notebook CircleLatticePoints.m.