|
|
|
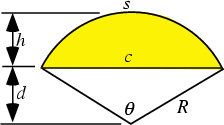
A portion of a Circle whose upper boundary is a circular Arc and whose lower boundary is a Chord making a
Central Angle ![]() radians (180°), illustrated above as the shaded region. Let
radians (180°), illustrated above as the shaded region. Let ![]() be the radius of the
Circle,
be the radius of the
Circle, ![]() the Chord length,
the Chord length, ![]() the Arc Length,
the Arc Length, ![]() the height of the arced portion, and
the height of the arced portion, and ![]() the height
of the triangular portion. Then
the height
of the triangular portion. Then
| (1) | |||
| (2) | |||
| (3) | |||
| (4) | |||
| (5) | |||
| (6) | |||
| (7) | |||
| (8) | |||
| (9) |
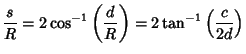 |
|||
| (10) |
| (11) | |||
| (12) | |||
| (13) | |||
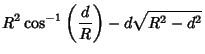 |
(14) | ||
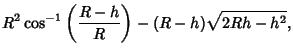 |
(15) |
See also Chord, Circle-Circle Intersection, Cylindrical Segment, Lens, Parabolic Segment, Sagitta, Sector, Spherical Segment
References
Beyer, W. H. (Ed.) CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press,
p. 125, 1987.
|
|
|
© 1996-9 Eric W. Weisstein