|
|
|
An analog of a Determinant where all the signs in the expansion by Minors are taken as Positive. The
permanent of a Matrix A is the coefficient of ![]() in
in
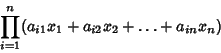
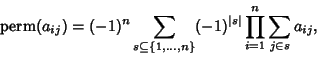
If
![]() is a Unitary Matrix, then
is a Unitary Matrix, then
See also Determinant, Frobenius-König Theorem, Immanant, Ryser Formula, Schur Matrix
References
Borovskikh, Y. V. and Korolyuk, V. S. Random Permanents. Philadelphia, PA: Coronet Books, 1994.
Minc, H. Permanents. Reading, MA: Addison-Wesley, 1978.
Vardi, I. ``Permanents.'' §6.1 in Computational Recreations in Mathematica.
Reading, MA: Addison-Wesley, pp. 108 and 110-112, 1991.