|
|
|
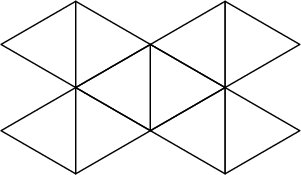
The pentagonal dipyramid is one of the convex Deltahedra, and Johnson Solid ![]() . It is also
the Dual Polyhedron of the Pentagonal Prism. The distance between two adjacent Vertices on the base of the Pentagon is
. It is also
the Dual Polyhedron of the Pentagonal Prism. The distance between two adjacent Vertices on the base of the Pentagon is
![$\displaystyle [1-{\textstyle{1\over 4}}(\sqrt{5}-1)]^2+\left[{(1+\sqrt{5})\sqrt{5-\sqrt{5}}\over 4\sqrt{2}\,}\right]^2$](p1_1145.gif) |
|||
| (1) |
| (2) |
| (3) |
| (4) |
| (5) |
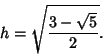 |
(6) |
| (7) |
See also Deltahedron, Dipyramid, Golden Mean, Icosahedron, Johnson Solid, Triangular Dipyramid