|
|
|
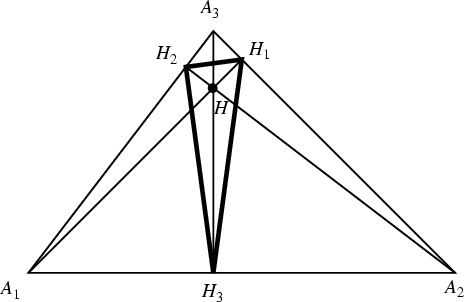
Given a Triangle
![]() ,
the Triangle
,
the Triangle
![]() with Vertices
at the feet of the Altitudes
(perpendiculars from a point to the sides) is called the orthic triangle. The three
lines
with Vertices
at the feet of the Altitudes
(perpendiculars from a point to the sides) is called the orthic triangle. The three
lines ![]() are Concurrent at the Orthocenter
are Concurrent at the Orthocenter ![]() of
of
![]() .
.
The centroid of the orthic triangle has Triangle Center Function
See also Altitude, Fagnano's Problem, Orthocenter, Pedal Triangle, Schwarz's Triangle Problem, Symmedian Point
References
Casey, J. A Treatise on the Analytical Geometry of the Point, Line, Circle, and Conic Sections, Containing
an Account of Its Most Recent Extensions, with Numerous Examples, 2nd ed., rev. enl. Dublin: Hodges, Figgis, & Co., p. 9, 1893.
Coxeter, H. S. M. and Greitzer, S. L. Geometry Revisited. Washington, DC: Math. Assoc. Amer., pp. 9 and 16-18,
1967.
Kimberling, C. ``Central Points and Central Lines in the Plane of a Triangle.'' Math. Mag. 67, 163-187, 1994.