|
|
|
| (1) |
| (2) |
| (3) |
| (4) |
| (5) |
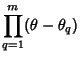 |
(6) | ||
| (7) | |||
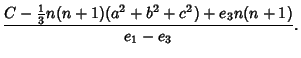 |
|||
| (8) |
References
Byerly, W. E. An Elementary Treatise on Fourier's Series, and Spherical, Cylindrical, and Ellipsoidal Harmonics,
with Applications to Problems in Mathematical Physics. New York: Dover, 1959.
Whittaker, E. T. and Watson, G. N. A Course in Modern Analysis, 4th ed. Cambridge, England:
Cambridge University Press, 1990.