|
|
|
The vector identity
| (1) |
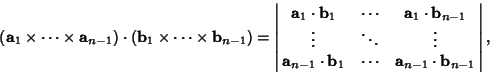 |
(2) |
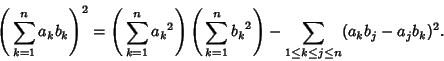 |
(3) |
See also Vector Triple Product, Vector Quadruple Product
References
Gradshteyn, I. S. and Ryzhik, I. M. Tables of Integrals, Series, and Products, 5th ed. San Diego, CA:
Academic Press, p. 1093, 1979.