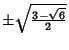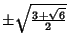Also called Hermite Quadrature. A Gaussian Quadrature over the interval
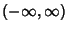 with
Weighting Function
with
Weighting Function 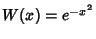 . The Abscissas for quadrature order
. The Abscissas for quadrature order 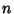 are given by the
roots of the Hermite Polynomials
are given by the
roots of the Hermite Polynomials 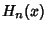 , which occur symmetrically about 0. The
Weights are
, which occur symmetrically about 0. The
Weights are
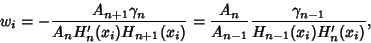 |
(1) |
where 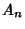 is the Coefficient of
is the Coefficient of 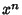 in
in 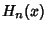 . For Hermite Polynomials,
. For Hermite Polynomials,
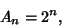 |
(2) |
so
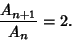 |
(3) |
Additionally,
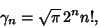 |
(4) |
so
Using the Recurrence Relation
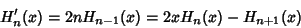 |
(6) |
yields
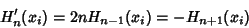 |
(7) |
and gives
![\begin{displaymath}
w_i={2^{n+1}n!\sqrt{\pi}\over[H_n'(x_i)]^2}={2^{n+1}n!\sqrt{\pi}\over[H_{n+1}(x_i)]^2}.
\end{displaymath}](h_1363.gif) |
(8) |
The error term is
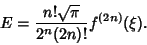 |
(9) |
Beyer (1987) gives a table of Abscissas and weights up to 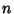 =12.
=12.
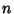 |
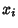 |
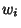 |
| 2 |
± 0.707107 |
0.886227 |
| 3 |
0 |
1.18164 |
| |
± 1.22474 |
0.295409 |
| 4 |
± 0.524648 |
0.804914 |
| |
± 1.65068 |
0.0813128 |
| 5 |
0 |
0.945309 |
| |
± 0.958572 |
0.393619 |
| |
± 2.02018 |
0.0199532 |
The Abscissas and weights can be computed analytically for small 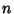 .
.
References
Beyer, W. H. CRC Standard Mathematical Tables, 28th ed. Boca Raton, FL: CRC Press, p. 464, 1987.
Hildebrand, F. B. Introduction to Numerical Analysis. New York: McGraw-Hill, pp. 327-330, 1956.
© 1996-9 Eric W. Weisstein
1999-05-25
![]() with
Weighting Function
with
Weighting Function ![]() . The Abscissas for quadrature order
. The Abscissas for quadrature order ![]() are given by the
roots of the Hermite Polynomials
are given by the
roots of the Hermite Polynomials ![]() , which occur symmetrically about 0. The
Weights are
, which occur symmetrically about 0. The
Weights are
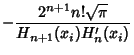
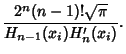
![]() .
.