Poisson's Equation equation is
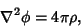 |
(1) |
where 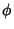 is often called a potential function and
is often called a potential function and 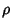 a density function, so the differential operator in this case is
a density function, so the differential operator in this case is
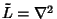 . As usual, we are looking for a Green's function
. As usual, we are looking for a Green's function
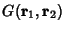 such that
such that
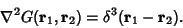 |
(2) |
But from Laplacian,
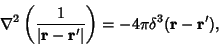 |
(3) |
so
 |
(4) |
and the solution is
![\begin{displaymath}
\phi({\bf r})=\int G({\bf r},{\bf r}')[4\pi \rho({\bf r}')]\...
... {\rho({\bf r}')\,d^3{\bf r}'\over\vert{\bf r}-{\bf r}'\vert}.
\end{displaymath}](g_2293.gif) |
(5) |
Expanding
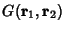 in the Spherical Harmonics
in the Spherical Harmonics 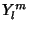 gives
gives
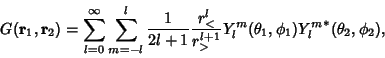 |
(6) |
where 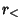 and
and 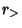 are Greater Than/Less Than Symbols. This expression simplifies
to
are Greater Than/Less Than Symbols. This expression simplifies
to
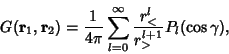 |
(7) |
where 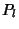 are Legendre Polynomials, and
are Legendre Polynomials, and
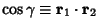 . Equations
(6) and (7) give the addition theorem for Legendre Polynomials.
. Equations
(6) and (7) give the addition theorem for Legendre Polynomials.
In Cylindrical Coordinates, the Green's function is much more complicated,
![\begin{displaymath}
G({\bf r}_1,{\bf r}_2) = {1\over 2\pi^2}\sum_{m=-\infty}^\in...
...\rho_<)K_m(k\rho_>)e^{im(\phi_1-\phi_2)}\cos[k(z_1-z_2)]\, dk,
\end{displaymath}](g_2301.gif) |
(8) |
where 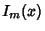 and
and 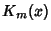 are Modified Bessel Functions of the First
and Second Kinds (Arfken 1985).
are Modified Bessel Functions of the First
and Second Kinds (Arfken 1985).
References
Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 485-486, 905, and 912, 1985.
© 1996-9 Eric W. Weisstein
1999-05-25
![]() in the Spherical Harmonics
in the Spherical Harmonics ![]() gives
gives
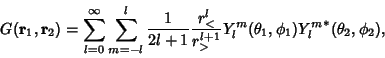
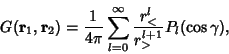
![\begin{displaymath}
G({\bf r}_1,{\bf r}_2) = {1\over 2\pi^2}\sum_{m=-\infty}^\in...
...\rho_<)K_m(k\rho_>)e^{im(\phi_1-\phi_2)}\cos[k(z_1-z_2)]\, dk,
\end{displaymath}](g_2301.gif)