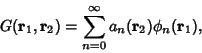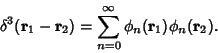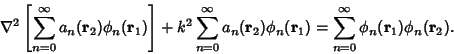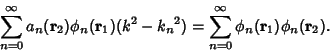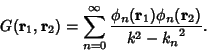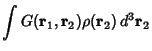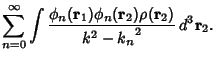The inhomogeneous Helmholtz Differential Equation is
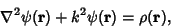 |
(1) |
where the Helmholtz operator is defined as
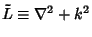 . The Green's function is then defined by
. The Green's function is then defined by
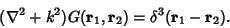 |
(2) |
Define the basis functions 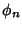 as the solutions to the homogeneous Helmholtz Differential Equation
as the solutions to the homogeneous Helmholtz Differential Equation
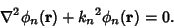 |
(3) |
The Green's function can then be expanded in terms of the 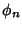 s,
s,
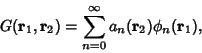 |
(4) |
and the Delta Function as
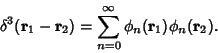 |
(5) |
Plugging (4) and (5) into (2) gives
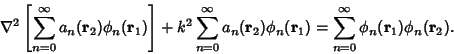 |
(6) |
Using (3) gives
 |
(7) |
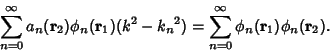 |
(8) |
This equation must hold true for each 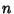 , so
, so
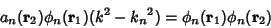 |
(9) |
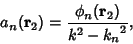 |
(10) |
and (4) can be written
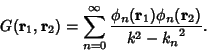 |
(11) |
The general solution to (1) is therefore
References
Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 529-530, 1985.
© 1996-9 Eric W. Weisstein
1999-05-25