|
|
|
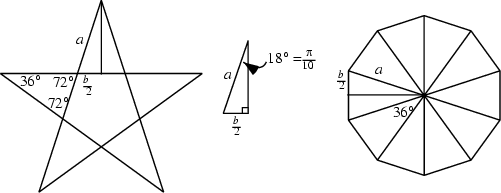
An Isosceles Triangle with Vertex angles 36°. Such Triangles
occur in the Pentagram and Decagon. The legs are in a Golden Ratio to the base. For such a
Triangle,
| (1) |
| (2) |
| (3) |
| (4) |
See also Decagon, Golden Ratio, Isosceles Triangle, Pentagram
References
Pappas, T. ``The Pentagon, the Pentagram & the Golden Triangle.'' The Joy of Mathematics.
San Carlos, CA: Wide World Publ./Tetra, pp. 188-189, 1989.