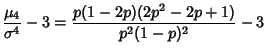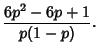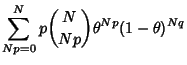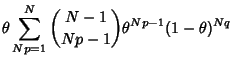A Distribution given by
The distribution of heads and tails in Coin Tossing is a Bernoulli distribution with 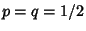 . The
Generating Function of the Bernoulli distribution is
. The
Generating Function of the Bernoulli distribution is
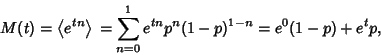 |
(3) |
so
and the Moments about 0 are
The Moments about the Mean are
The Mean, Variance, Skewness, and Kurtosis are then
To find an estimator for a population mean,
so
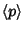 is an Unbiased Estimator for
is an Unbiased Estimator for 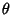 . The probability of
. The probability of 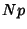 successes in
successes in 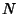 trials is then
trials is then
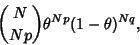 |
(19) |
where
![\begin{displaymath}
p={\hbox{[number of successes]}\over N} \equiv {n\over N}.
\end{displaymath}](b_510.gif) |
(20) |
See also Binomial Distribution
© 1996-9 Eric W. Weisstein
1999-05-26
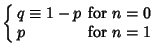
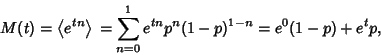
![$\displaystyle {\mu_3\over \sigma^3} = {p(1-p)(1-2p)\over [p(1-p)]^{3/2}}$](b_498.gif)