A total order satisfies the conditions for a Partial Order plus the comparability condition. A Relation
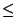 is a partial order on a Set
is a partial order on a Set 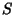 if
if
- 1. Reflexivity:
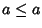 for all
for all 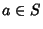
- 2. Antisymmetry:
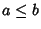 and
and 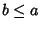 implies
implies 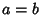
- 3. Transitivity:
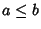 and
and 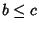 implies
implies 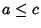 ,
,
and is a total order if, in addition,
- 4. Comparability: For any
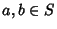 , either
, either 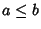 or
or 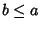 .
.
See also Partial Order, Relation
© 1996-9 Eric W. Weisstein
1999-05-26
![]() is a partial order on a Set
is a partial order on a Set ![]() if
if