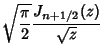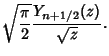Take the Helmholtz Differential Equation
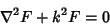 |
(1) |
in Spherical Coordinates. This is just Laplace's Equation in Spherical Coordinates with an additional
term,
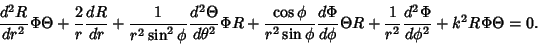 |
(2) |
Multiply through by
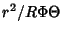 ,
,
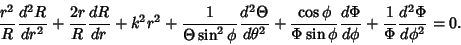 |
(3) |
This equation is separable in 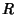 . Call the separation constant
. Call the separation constant 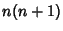 ,
,
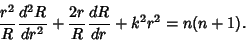 |
(4) |
Now multiply through by 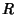 ,
,
![\begin{displaymath}
r^2{d^2R\over dr^2} + 2r{dR\over dr} + [k^2r^2-n(n+1)]R = 0.
\end{displaymath}](s2_854.gif) |
(5) |
This is the Spherical Bessel Differential Equation. It can be transformed by letting 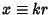 , then
, then
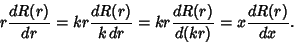 |
(6) |
Similarly,
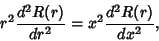 |
(7) |
so the equation becomes
![\begin{displaymath}
x^2{d^2R\over dx^2} + 2x{dR\over dx} + [x^2-n(n+1)]R = 0.
\end{displaymath}](s2_858.gif) |
(8) |
Now look for a solution of the form
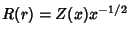 , denoting a derivative with respect to
, denoting a derivative with respect to 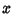 by a prime,
by a prime,
so
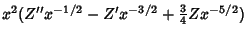
|
|
![$ +2x(Z'x^{-1/2}-{\textstyle{1\over 2}}Zx^{-3/2}) + [x^2-n(n+1)]Zx^{-1/2}=0$](s2_866.gif)
|
|
|
|
(11) |
![\begin{displaymath}
x^2(Z''-Z'x^{-1}+{\textstyle{3\over 4}}Zx^{-2})+2x(Z'-{\textstyle{1\over 2}}Zx^{-1})+[x^2-n(n+1)]Z=0
\end{displaymath}](s2_867.gif) |
(12) |
![\begin{displaymath}
x^2Z''+(-x+2x)Z'+[{\textstyle{3\over 4}}-1+x^2-n(n+1)]Z=0
\end{displaymath}](s2_868.gif) |
(13) |
![\begin{displaymath}
x^2Z''+xZ'+[x^2-(n^2+n+{\textstyle{1\over 4}})]Z=0
\end{displaymath}](s2_869.gif) |
(14) |
![\begin{displaymath}
x^2Z''+xZ'+[x^2-(n+{\textstyle{1\over 2}})^2]Z = 0.
\end{displaymath}](s2_870.gif) |
(15) |
But the solutions to this equation are Bessel Functions of half integral order, so the normalized
solutions to the original equation are
 |
(16) |
which are known as Spherical Bessel Functions. The two types of solutions are denoted
 (Spherical Bessel Function of the First Kind) or
(Spherical Bessel Function of the First Kind) or 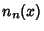 (Spherical Bessel Function of the Second
Kind), and the general solution is written
(Spherical Bessel Function of the Second
Kind), and the general solution is written
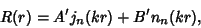 |
(17) |
where
See also Spherical Bessel Function, Spherical Bessel Function of the First Kind, Spherical Bessel Function of
the Second Kind
References
Abramowitz, M. and Stegun, C. A. (Eds.).
Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing.
New York: Dover, p. 437, 1972.
© 1996-9 Eric W. Weisstein
1999-05-26