|
|
|
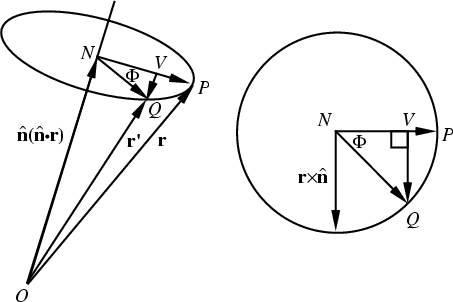
A formula which relates the Vector ![]() to the Angle
to the Angle ![]() in the above figure (Goldstein 1980). Referring to
the figure,
in the above figure (Goldstein 1980). Referring to
the figure,
See also Euler Angles, Euler Parameters
References
Goldstein, H. Classical Mechanics, 2nd ed. Reading, MA: Addison-Wesley, 1980.