|
|
|
For ![]() and using the Notation of the Ramanujan Theta Function,
the Rogers-Ramanujan identities are
and using the Notation of the Ramanujan Theta Function,
the Rogers-Ramanujan identities are
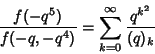 |
(1) |
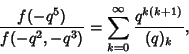 |
(2) |
| (3) |
| (4) |
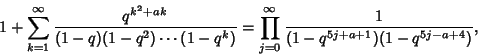 |
(5) |
Other forms of the Rogers-Ramanujan identities include
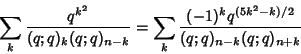 |
(6) |
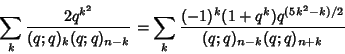 |
(7) |
See also Andrews-Schur Identity
References
Andrews, G. E. The Theory of Partitions. Cambridge, England: Cambridge University Press, 1985.
Andrews, G. E.
Andrews, G. E. and Baxter, R. J. ``A Motivated Proof of the Rogers-Ramanujan Identities.'' Amer. Math. Monthly 96, 401-409, 1989.
Bressoud, D. M. Analytic and Combinatorial Generalizations of the Rogers-Ramanujan Identities.
Providence, RI: Amer. Math. Soc., 1980.
Hardy, G. H. Ramanujan: Twelve Lectures on Subjects Suggested by His Life and Work, 3rd ed. New York: Chelsea, p. 13, 1959.
Paule, P. ``Short and Easy Computer Proofs of the Rogers-Ramanujan Identities and of Identities of Similar Type.'' Electronic J. Combinatorics 1, R10 1-9, 1994.
http://www.combinatorics.org/Volume_1/volume1.html#R10.
Petkovsek, M.; Wilf, H. S.; and Zeilberger, D. A=B. Wellesley, MA: A. K. Peters, p. 117, 1996.
Robinson, R. M. ``Comment to: `A Motivated Proof of the Rogers-Ramanujan Identities.''' Amer. Math. Monthly 97, 214-215, 1990.
Rogers, L. J. ``Second Memoir on the Expansion of Certain Infinite Products.'' Proc. London Math. Soc. 25, 318-343, 1894.
Sloane, N. J. A. Sequence
A006141/M0260
in ``An On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html and Sloane, N. J. A. and Plouffe, S.
The Encyclopedia of Integer Sequences. San Diego: Academic Press, 1995.
![]() -Series: Their Development and Application in Analysis, Number Theory, Combinatorics, Physics, and Computer Algebra.
Providence, RI: Amer. Math. Soc., pp. 17-20, 1986.
-Series: Their Development and Application in Analysis, Number Theory, Combinatorics, Physics, and Computer Algebra.
Providence, RI: Amer. Math. Soc., pp. 17-20, 1986.
|
|
|
© 1996-9 Eric W. Weisstein