If 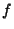 is a continuous function that satisfies the Lipschitz Condition
is a continuous function that satisfies the Lipschitz Condition
in a surrounding of
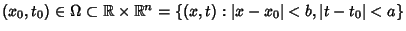 , then the
differential equation
, then the
differential equation
has a unique solution 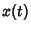 in the interval
in the interval 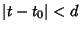 , where
, where
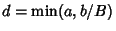 , min denotes the Minimum,
, min denotes the Minimum,
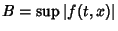 , and sup denotes the Supremum.
, and sup denotes the Supremum.
See also Ordinary Differential Equation
© 1996-9 Eric W. Weisstein
1999-05-25
![]() is a continuous function that satisfies the Lipschitz Condition
is a continuous function that satisfies the Lipschitz Condition