- 1. If
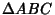 and
and 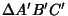 are two directly similar triangles, while
are two directly similar triangles, while 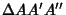 ,
, 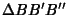 , and
, and
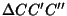 are three directly similar triangles, then
are three directly similar triangles, then
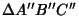 is directly similar to
is directly similar to 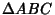 .
.
- 2. When all the points
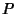 on
on 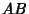 are related by a Similarity Transformation to all the points
are related by a Similarity Transformation to all the points 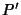 on
on 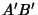 ,
the points dividing the segment
,
the points dividing the segment 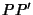 in a given ratio are distant and collinear, or else they coincide.
in a given ratio are distant and collinear, or else they coincide.
References
Coxeter, H. S. M. and Greitzer, S. L. Geometry Revisited. Washington, DC: Math. Assoc. Amer., pp. 95-100, 1967.
© 1996-9 Eric W. Weisstein
1999-05-26