|
|
|
Let ![]() be the sum of the products of distinct Roots
be the sum of the products of distinct Roots ![]() of the Polynomial equation of degree
of the Polynomial equation of degree ![]()
| (1) |
| (2) | |||
| (3) | |||
| (4) |
| (5) |
| (6) |
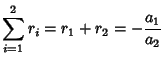 |
(7) | ||
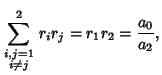 |
(8) |
|
|
|
|
|
(9) |
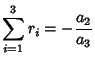 |
(10) | ||
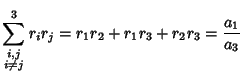 |
(11) | ||
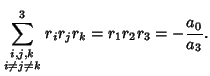 |
(12) |
See also Elementary Symmetric Function
References
Coolidge, J. L. A Treatise on Algebraic Plane Curves. New York: Dover, pp. 1-2, 1959.
|
|
|
© 1996-9 Eric W. Weisstein