If there are two functions 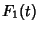 and
and  with the same integral transform
with the same integral transform
![\begin{displaymath}
{\mathcal T}[F_1(t)] = {\mathcal T}[F_2(t)] \equiv f(s),
\end{displaymath}](l1_1639.gif) |
(1) |
then a Null Function can be defined by
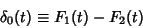 |
(2) |
so that the integral
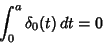 |
(3) |
vanishes for all 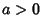 .
.
See also Null Function
© 1996-9 Eric W. Weisstein
1999-05-26
![]() and
and ![]() with the same integral transform
with the same integral transform