|
|
|
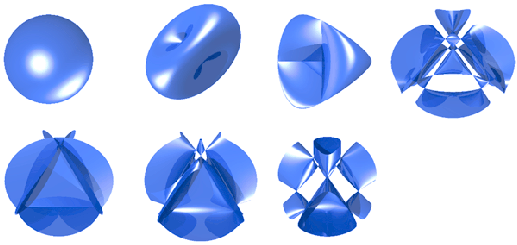
The Kummer surfaces are a family of Quartic Surfaces given by the algebraic equation
| (1) |
| (2) |
| (3) | |||
| (4) | |||
| (5) | |||
| (6) |
| (7) |
| (8) |
| (9) |
The following table gives the number of Ordinary Double Points for various ranges of ![]() ,
corresponding to the preceding illustrations.
,
corresponding to the preceding illustrations.
|
|
4 | 12 |
|
|
||
|
|
4 | 12 |
| 16 | 0 | |
| 16 | 0 |
The Kummer surfaces can be represented parametrically by hyperelliptic Theta Functions. Most of the Kummer surfaces admit 16 Ordinary Double Points, the maximum possible for a Quartic Surface. A special case of a Kummer surface is the Tetrahedroid.
Nordstrand gives the implicit equations as
| (10) |
| (11) |
See also Quartic Surface, Roman Surface, Tetrahedroid
References
Endraß, S. ``Flächen mit vielen Doppelpunkten.'' DMV-Mitteilungen 4, 17-20, Apr. 1995.
Endraß, S. ``Kummer Surfaces.''
http://www.mathematik.uni-mainz.de/AlgebraischeGeometrie/docs/Ekummer.shtml.
Fischer, G. (Ed.). Mathematical Models from the Collections of Universities and Museums.
Braunschweig, Germany: Vieweg, pp. 14-19, 1986.
Fischer, G. (Ed.). Plates 34-37 in
Mathematische Modelle/Mathematical Models, Bildband/Photograph Volume.
Braunschweig, Germany: Vieweg, pp. 33-37, 1986.
Guy, R. K. Unsolved Problems in Number Theory, 2nd ed. New York: Springer-Verlag, p. 183, 1994.
Hudson, R. Kummer's Quartic Surface. Cambridge, England: Cambridge University Press, 1990.
Kummer, E. ``Über die Flächen vierten Grades mit sechszehn singulären Punkten.'' Ges. Werke 2, 418-432.
Kummer, E. ``Über Strahlensysteme, deren Brennflächen Flächen vierten Grades mit sechszehn singulären Punkten sind.''
Ges. Werke 2, 418-432.
Nordstrand, T. ``Kummer's Surface.''
http://www.uib.no/people/nfytn/kummtxt.htm.
|
|
|
© 1996-9 Eric W. Weisstein