|
|
|
An initial value problem is a problem that has its conditions specified at some time ![]() . Usually, the problem is an
Ordinary Differential Equation or a Partial Differential Equation. For example,
. Usually, the problem is an
Ordinary Differential Equation or a Partial Differential Equation. For example,
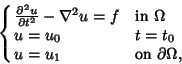
See also Boundary Conditions, Boundary Value Problem, Partial Differential Equation
References
Eriksson, K.; Estep, D.; Hansbo, P.; and Johnson, C. Computational Differential Equations.
Lund, Sweden: Studentlitteratur, 1996.