|
|
|
Let
![]() be an
be an ![]() Determinant with Complex (or Real)
elements
Determinant with Complex (or Real)
elements ![]() , then
, then
![]() if
if
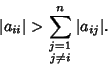
See also Hadamard's Inequality
References
Gradshteyn, I. S. and Ryzhik, I. M. Tables of Integrals, Series, and Products, 5th ed. San Diego, CA:
Academic Press, p. 1110, 1979.