Direct Product (Tensor)

For a first-Rank Tensor (i.e., a Vector),

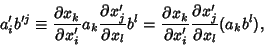$$a_i'b'^j \equiv {\partial x_k\over\partial x_i'} a_k {\parti... ...ver \partial x_i'} {\partial x_j'\over \partial x_l} (a_kb^l),$$ (1)

which is a second-Rank Tensor. The Contraction of a direct product of first-Rank Tensors is the Scalar

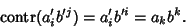$$\mathop{\rm contr}(a_i'b'^j) = a_i'b'^i = a_kb^k.$$ (2)

For a second-Rank Tensor,

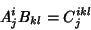$$A^i_jB_{kl} = C_j^{ikl}$$ (3)

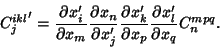$${C_j^{ikl}}' = {\partial x_i'\over \partial x_m} {\partial x... ...ver \partial x_p} {\partial x_l'\over \partial x_q} C_n^{mpq}.$$ (4)

For a general Tensor, the direct product of two Tensors is a Tensor of Rank equal to the sum of the two initial Ranks. The direct product is Associative, but not Commutative.

References

Arfken, G. ``Contraction, Direct Product.'' §3.2 in Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 124-126, 1985.