|
|
|
A continued fraction with partial quotients which increase in Arithmetic Progression is
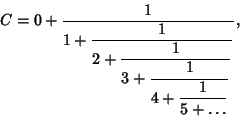
See also e, Golden Mean, Modified Bessel Function of the First Kind, Pi, Rabbit Constant, Thue-Morse Constant
References
Beeler, M.; Gosper, R. W.; and Schroeppel, R. HAKMEM. Cambridge, MA: MIT Artificial Intelligence Laboratory, Memo AIM-239, Feb. 1972.
Finch, S. ``Favorite Mathematical Constants.'' http://www.mathsoft.com/asolve/constant/cntfrc/cntfrc.html
Guy, R. K. ``Review: The Mathematics of Plato's Academy.'' Amer. Math. Monthly 97, 440-443, 1990.
Lehmer, D. H. ``Continued Fractions Containing Arithmetic Progressions.'' Scripta Math. 29, 17-24, 1973.
Rabinowitz, S. Problem E3264. ``Asymptotic Estimates from Convergents of a Continued Fraction.''
Amer. Math. Monthly 97, 157-159, 1990.