A pair of Positive Integers 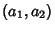 such that the equations
such that the equations
have a Positive Integer solution 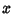 , where
, where 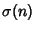 is the Divisor Function. If
is the Divisor Function. If 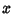 is Prime, then
is Prime, then
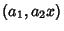 is an Amicable Pair (te Riele 1986).
is an Amicable Pair (te Riele 1986). 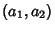 is a ``special'' breeder if
is a ``special'' breeder if
where 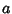 and
and 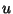 are Relatively Prime,
are Relatively Prime, 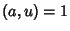 . If regular amicable pairs of type
. If regular amicable pairs of type 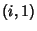 with
with 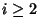 are
of the form
are
of the form 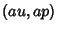 with
with 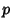 Prime, then
Prime, then 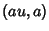 are special breeders (te Riele 1986).
are special breeders (te Riele 1986).
References
te Riele, H. J. J. ``Computation of All the Amicable Pairs Below 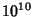 .'' Math. Comput. 47, 361-368 and S9-S35, 1986.
.'' Math. Comput. 47, 361-368 and S9-S35, 1986.
© 1996-9 Eric W. Weisstein
1999-05-26
![]() such that the equations
such that the equations
![]() .'' Math. Comput. 47, 361-368 and S9-S35, 1986.
.'' Math. Comput. 47, 361-368 and S9-S35, 1986.