|
|
|
Let ![]() and
and ![]() ,
, ![]() , ...be the Positive Roots of
, ...be the Positive Roots of ![]() . An expansion of a function in
the interval (0,1) in terms of Bessel Functions of the First Kind
. An expansion of a function in
the interval (0,1) in terms of Bessel Functions of the First Kind
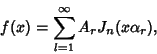 |
(1) |
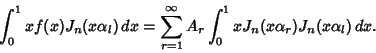 |
(2) |
| (3) |
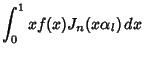 |
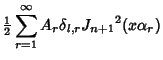 |
||
| (4) |
| (5) |
References
Bowman, F. Introduction to Bessel Functions. New York: Dover, 1958.