|
|
|
The following Integral Transform relationship, known as the Abel transform, exists between two functions ![]() and
and
![]() for
for ![]() ,
,
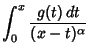 |
(1) | ||
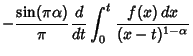 |
(2) | ||
![$\displaystyle -{\sin(\pi\alpha)\over\pi}\left[{\int_0^t{df\over dx} {dx\over(t-x)^{1-\alpha}} + {f(0)\over t^{1-\alpha}}}\right].$](a_99.gif) |
(3) |
References
Arfken, G. Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, pp. 875-876, 1985.
Binney, J. and Tremaine, S. Galactic Dynamics. Princeton, NJ: Princeton University Press, p. 651, 1987.
Bracewell, R. The Fourier Transform and Its Applications. New York: McGraw-Hill, pp. 262-266, 1965.