|
|
|
The sequence of numbers given by
| (1) |
| (2) |
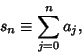 |
(3) |
| (4) |
References
Blecksmith, R. and Laud, P. W. ``Some Exact Number Theory Computations via Probability Mechanisms.''
Amer. Math. Monthly 102, 893-903, 1995.
Brillhart, J.; Erdös, P.; and Morton, P. ``On the Sums of the Rudin-Shapiro Coefficients II.'' Pac. J. Math.
107, 39-69, 1983.
Brillhart, J. and Morton, P. ``Über Summen von Rudin-Shapiroschen Koeffizienten.'' Ill. J. Math. 22,
126-148, 1978.
France, M. M. and van der Poorten, A. J. ``Arithmetic and Analytic Properties of Paper Folding Sequences.''
Bull. Austral. Math. Soc. 24, 123-131, 1981.