A Partial Differential Equation of second-order, i.e., one of the form
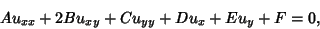 |
(1) |
is called parabolic if the Matrix
![\begin{displaymath}
{\hbox{\sf Z}} \equiv \left[{\matrix{A & B\cr B & C\cr}}\right]
\end{displaymath}](p1_456.gif) |
(2) |
satisfies det
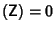 . The Heat Conduction Equation and other
diffusion equations
. The Heat Conduction Equation and other
diffusion equations  are examples. Initial-boundary conditions are used to give
are examples. Initial-boundary conditions are used to give
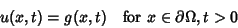 |
(3) |
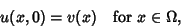 |
(4) |
where
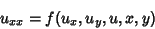 |
(5) |
holds in 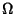 .
.
See also Elliptic Partial Differential Equation, Hyperbolic Partial Differential Equation,
Partial Differential Equation
© 1996-9 Eric W. Weisstein
1999-05-26