|
|
|
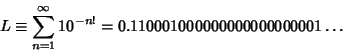
See also Liouville Number
References
Conway, J. H. and Guy, R. K. ``Liouville's Number.'' In The Book of Numbers. New York: Springer-Verlag,
pp. 239-241, 1996.
Courant, R. and Robbins, H. ``Liouville's Theorem and the Construction of Transcendental Numbers.'' §2.6.2 in
What is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed.
Oxford, England: Oxford University Press, pp. 104-107, 1996.
Liouville, J. ``Sur des classes très étendues de quantités dont la valeur n'est ni algébrique, ni
même reductible à des irrationelles algébriques.'' C. R. Acad. Sci. Paris 18, 883-885 and 993-995, 1844.
Liouville, J. ``Sur des classes très-étendues de quantités dont la valeur n'est ni algébrique, ni même réductible à des
irrationelles algébriques.'' J. Math. pures appl. 15, 133-142, 1850.
Sloane, N. J. A. Sequence
A012245
in ``The On-Line Version of the Encyclopedia of Integer Sequences.''
http://www.research.att.com/~njas/sequences/eisonline.html.