|
|
|
The function defined by
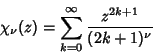
See also Polylogarithm
References
Cvijovic, D. and Klinowski, J. ``Closed-Form Summation of Some Trigonometric Series.'' Math. Comput. 64, 205-210, 1995.
Lewin, L. Polylogarithms and Associated Functions. Amsterdam, Netherlands: North-Holland, pp. 282-283, 1981.