|
|
|
The expected number of Real zeros ![]() of a Random Polynomial of degree
of a Random Polynomial of degree ![]() is
is
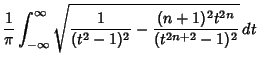 |
(1) | ||
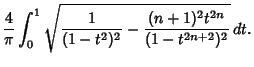 |
(2) |
| (3) |
![\begin{displaymath}
C_1={2\over\pi}\left[{\ln 2+\int_0^\infty \left({\sqrt{{1\ov...
...)^2}-{1\over x+1}}\,\,}\right)\,dx}\right]=0.6257358072\ldots.
\end{displaymath}](k_208.gif) |
(4) |
References
Edelman, A. and Kostlan, E. ``How Many Zeros of a Random Polynomial are Real?'' Bull. Amer. Math. Soc. 32, 1-37, 1995.
Kac, M. ``On the Average Number of Real Roots of a Random Algebraic Equation.'' Bull. Amer. Math. Soc. 49, 314-320, 1943.
Kac, M. ``A Correction to `On the Average Number of Real Roots of a Random Algebraic Equation'.'' Bull. Amer. Math. Soc.
49, 938, 1943.